The length of
 in the triangle is 9 units. Therefore, the correct answer from the provided options is 9.
in the triangle is 9 units. Therefore, the correct answer from the provided options is 9.
In a 45°-45°-90° triangle, the sides have a specific ratio: the length of the hypotenuse is
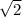 times the length of each of the other two sides, which are of equal length. This type of triangle is sometimes referred to as an isosceles right triangle.
times the length of each of the other two sides, which are of equal length. This type of triangle is sometimes referred to as an isosceles right triangle.
Given that the hypotenuse is
 and we need to find the length of one of the legs (x), we can use the relationship:
and we need to find the length of one of the legs (x), we can use the relationship:
![\[ \text{Hypotenuse} = x√(2) \]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/epyz8gybdjxatgpokctj3gnazsjtip44kd.png)
![\[ 9√(2) = x√(2) \]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/l9slc84bgfxw4iuuks0ijzlj4dr81yiexy.png)
Solving for x:
![\[ x = (9√(2))/(√(2)) \]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/72a4dtkgir69d2cm4sxyj387cb74tmb14v.png)
![\[ x = 9 \]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/cxbmv163lcqrpv2pilsrgkh863je4pbhtv.png)
The length of
 in the triangle is 9 units. Therefore, the correct answer from the provided options is 9.
in the triangle is 9 units. Therefore, the correct answer from the provided options is 9.