Answer:
$20,086.35
Explanation:
To calculate the maturity value by compound interest, we will use the formula
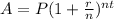
where,
A = Maturity amount
P = Principal amount = $10,000
r = rate of interest = 4.65% = 0.0465
n = number of compounding periods = 365
t = time in years = 15 years
Now substituting the values,

=
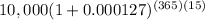
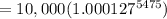
= 10,000(2.008635)
= 20086.353758 ≈ $20,086.35
The final value of your investment would be $20,086.35.