Answer:
We conclude that there is a difference in the proportion of vines infested using Pernod 5 as opposed to Action.
Explanation:
We are given that Two new insecticides have just been marketed: Pernod 5 and Action. The results are:
Insecticide Number of Vines Checked (sample size) Number of Infested Vines
Pernod 5 400 26
Action 380 40
Let
 = proportion of vines infested using Pernod 5.
= proportion of vines infested using Pernod 5.
 = proportion of vines infested using Action.
= proportion of vines infested using Action.
So, Null Hypothesis,
 :
:
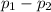 = 0 {means that there is no difference in the proportion of vines infested using Pernod 5 as opposed to Action}
= 0 {means that there is no difference in the proportion of vines infested using Pernod 5 as opposed to Action}
Alternate Hypothesis,
 :
:
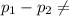 0 {means that there is a difference in the proportion of vines infested using Pernod 5 as opposed to Action}
0 {means that there is a difference in the proportion of vines infested using Pernod 5 as opposed to Action}
The test statistics that would be used here Two-sample z proportion statistics;
T.S. =
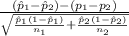 ~ N(0,1)
~ N(0,1)
where,
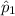 = sample proportion of vines infested using Pernod 5 =
= sample proportion of vines infested using Pernod 5 =
 = 0.065
= 0.065
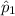 = sample proportion of vines infested using Action =
= sample proportion of vines infested using Action =
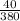 = 0.105
= 0.105
 = sample of Pernod 5 vines = 400
= sample of Pernod 5 vines = 400
 = sample of Action vines = 380
= sample of Action vines = 380
So, the test statistics =
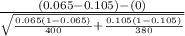
= -2.002
The value of z test statistics is -2.002.
Now, at 0.05 significance level the z table gives critical values of -1.96 and 1.96 for two-tailed test.
Since our test statistics does not lie within the range of critical values of z, so we have sufficient evidence to reject our null hypothesis as it will fall in the rejection region due to which we reject our null hypothesis.
Therefore, we conclude that there is a difference in the proportion of vines infested using Pernod 5 as opposed to Action.