Answer:
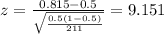
We can calculate the p value using the fact that we are conducting a right tailed test:

Since the p value i approximately 0 we can conclude that we have enough evidence to say that with this method, the probability of a baby being a boy is significantly greater than 0.5
Explanation:
Information given
n=172+39=211 represent the sample size
X=172 represent the number of boys in the sample
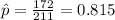 estimated proportion of of boys
estimated proportion of of boys
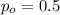 is the value to verify
is the value to verify
z would represent the statistic
 represent the p value
represent the p value
Hypothesis to test
The ides is verify if with this method, the probability of a baby being a boy is greater than 0.5, so then the system of hypothesis are.:
Null hypothesis:
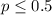
Alternative hypothesis:
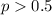
The statitic is given by:
 (1)
(1)
Replacing the info we got:
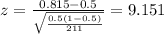
We can calculate the p value using the fact that we are conducting a right tailed test:

Since the p value i approximately 0 we can conclude that we have enough evidence to say that with this method, the probability of a baby being a boy is significantly greater than 0.5