Question

Kiley gathered the data in the table. She found the approximate line of best fit to be y = 1.6x – 4. A 2-column table with 5 rows. The first column is labeled x with entries 0, 2, 3, 5, 6. The second column is labeled y with entries negative 3, negative 1, negative 1, 5, 6. What is the residual value when x = 3? –1.8 –0.2 0.2 1.8

Answer 1

The residual value is -1.8 when x = 3

Explanation:

We are given the following table

x | y

0 | -3

2 | -1

3 | -1

5 | 5

6 | 6

Residual value:

A residual value basically shows the position of a data point with respect to the line of best fit.

The residual value is calculated as,

Residual value = Observed value - Predicted value

Where observed values are already given in the question and the predicted values are calculated by using the equation of line of best fit.

`y = 1.6x - 4`

When we substitute x = 3 in the above equation then we would get the predicted value.

`y = 1.6x - 4 \\\\y = 1.6(3) - 4 \\\\y = 4.8 - 4 \\\\y = 0.8`

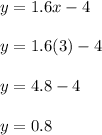
So the predicted value is 0.8

From the given table, the observed value corresponding to x = 3 is -1

So the residual value is,

Residual value = Observed value - Predicted value

Residual value = -1 - 0.8

Residual value = -1.8

Therefore, the residual value is -1.8 when x = 3

Note: A residual value closer to 0 is desired which means that the regression line best fits the data.

Answer 2

–1.8

Explanation:

Given the following table:

x y

0 -3

2 -1

3 -1

5 5

6 6

We are also given y = 1.6x – 4

Therefore, when x = 3, we have

Predicted y = 1.6(3) – 4 = 0.8

Since the observed y = - 1 when x = 3 on the table, the residual value can be estimated as follows:

Residual value = Observed value of y - Predicted value of y = -1 - 0.8 = - 1.8.

Therefore, the residual value when x = 3 is –1.8.