Question

If the diameter of a smaller circle is 7 km and the width of the circular path is 0.5 km, find the area of the path.

Answer 1

We have been given that the diameter of a smaller circle is 7 km and the width of the circular path is 0.5 km. We are asked to find the area of the path.

The area of the path will be equal to area of larger circle minus area of smaller circle.

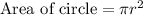`\text{Area of circle}=\pi r^2`, where r represents radius of circle.

We know that radius is half the diameter, so radius of smaller circle would be
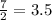`(7)/(2)=3.5` km.

The radius of the larger circle will be radius of smaller circle plus width of the path that is
`3.5+0.5=4` km.

`\text{Area of circular path}=\pi (4)^2-\pi (3.5)^2`

`\text{Area of circular path}=16\pi-12.25\pi`

`\text{Area of circular path}=3.75\pi`

`\text{Area of circular path}=11.78097`

`\text{Area of circular path}\approx 11.8`

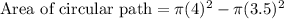
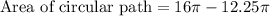
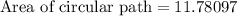
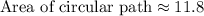
Therefore, the area of the circular path is approximately 11.8 square km.