Question

Odds and probability describe the same thing but in different ways. A.) true B.) false

Answer 1

True

Explanation:

I tried false and got it wrong.

Answer 2

The correct option is (B) False.

Explanation:

The probability of an event taking place is the portion of times that event is expected to be seen in many trials.

Consider an event E. The probability of the event E is given as follows:

`P(E)=(n(E))/(N)`

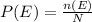
Here,

n (E) = Favorable outcomes

N = total outcomes.

Probability of an event ranges between 0 and 1.

The odds are defined as the ratio of the probability that the event will occur to the probability that the event will not occur.

The odds for the event E can be defined as follows:

`\text{Odds of event E}=(P(E))/(1-P(E))`

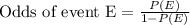
Thus, the statement provided: "Odds and probability describe the same thing but in different ways" is not true.

The correct option is (B).