1 Answer

Rowhawn · Answer 1 · 2021-01-20T17:42:52+0000

We have been given a triangular prism. We are asked to find the volume of a prism that is 3 times as large.

The sides of base of triangular prism and height of new prism would be 3 times the sides and height of original prism.

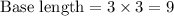
$\text{Base length}=3* 3=9$

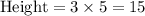
$\text{Height}=3* 5=15$

We can see that base of prism is a right triangle, so base length will be 9 and height of triangle will be 9 as well.

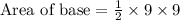
$\text{Area of base}=(1)/(2)* 9* 9$

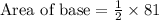
$\text{Area of base}=(1)/(2)* 81$

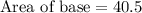
$\text{Area of base}=40.5$

Volume of prism would be base area times height of prism.

V=bh

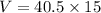
V=40.5* 15

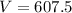
V=607.5

Therefore, the volume of the prism that is 3 times as large as given prism would be 607.5 and option D is the correct choice.

Please log in or register to add a comment.