Answer:
The given function is
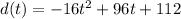
This function models the distance the quarter is above the water.
In this case, the x-axis represents the water level.
(a)
The coin was tossed at 112 feet, because that's the initial valur of the given function. Notice that the constant term is 112, which doesn't depend on any variable, that means it's a number that won't change, because that's the initial height.
(b)
To find the maximum height, we need to find the vertex of the function, which has coordinates of
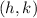 , where
, where
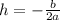 and
and
 . Also, from the equation we know
. Also, from the equation we know
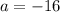 ,
,
 and
and
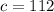 .
.
Using these values, we can find the vertex


The maximum height is 256 feet, and the time needed to reach it was 3 seconds.
(c)
As we found before, the maximum height is 256 feet.
(d)
After 6 seconds the quarter will hit the water. This answer is found by deduction only, because, if the maximum height took 3 seconds, then it would take 3 seconds more to hit the water.