Question

A recent study reports that women trust recommendations from Linkedin more than recommendations from any other social network platform. The question that arises is that whether trust in Linkedin recomendations differ by gender? The data on this worksheet shows the number of women and men who expressed that they trust recommendations made on LinkedIn in a recent survey.

Provide a 95% confidence interval estimate of the difference between the proportion of women and men who trust recommendations made on LinkedIn.
Women Men
Sample size 150 170
Trust Recommendations Made on LinkedIn 117 102

Answer 1

95% confidence interval estimate of the difference between the proportion of women and men who trust recommendations made on LinkedIn is [0.081 , 0.279].

Explanation:

We are given the data that shows the number of women and men who expressed that they trust recommendations made on LinkedIn in a recent survey;

Gender Women Men

Sample size 150 170

Trust Recommendations Made on LinkedIn 117 102

Firstly, the pivotal quantity for 95% confidence interval for the difference between population proportions is given by;

P.Q. =
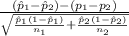`\frac{(\hat p_1-\hat p_2)-(p_1-p_2)}{\sqrt{(\hat p_1(1-\hat p_1))/(n_1)+(\hat p_2(1-\hat p_2))/(n_2) } }` ~ N(0,1)

where,
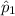`\hat p_1` = sample proportion of women who trust recommendations made on LinkedIn =
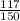`(117)/(150)` = 0.78

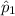`\hat p_1` = sample proportion of men who trust recommendations made on LinkedIn =
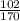`(102)/(170)` = 0.60

`n_1` = sample of women = 150

`n_2` = sample of men = 170

Here for constructing 95% confidence interval we have used Two-sample z proportion statistics.

So, 95% confidence interval for the difference between population proportions, (
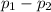`p_1-p_2`) is ;

P(-1.96 < N(0,1) < 1.96) = 0.95 {As the critical value of z at 2.5% level

of significance are -1.96 & 1.96}

P(-1.96 <
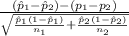`\frac{(\hat p_1-\hat p_2)-(p_1-p_2)}{\sqrt{(\hat p_1(1-\hat p_1))/(n_1)+(\hat p_2(1-\hat p_2))/(n_2) } }` < 1.96) = 0.95

P(
`-1.96 * {\sqrt{(\hat p_1(1-\hat p_1))/(n_1)+(\hat p_2(1-\hat p_2))/(n_2) } }` <
`{(\hat p_1-\hat p_2)-(p_1-p_2)}` <
`1.96 * {\sqrt{(\hat p_1(1-\hat p_1))/(n_1)+(\hat p_2(1-\hat p_2))/(n_2) } }` ) = 0.95

P(
`(\hat p_1-\hat p_2)-1.96 * {\sqrt{(\hat p_1(1-\hat p_1))/(n_1)+(\hat p_2(1-\hat p_2))/(n_2) } }` < (
`p_1-p_2`) <
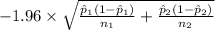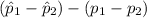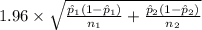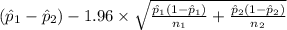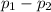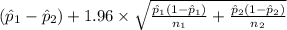`(\hat p_1-\hat p_2)+1.96 * {\sqrt{(\hat p_1(1-\hat p_1))/(n_1)+(\hat p_2(1-\hat p_2))/(n_2) } }` ) = 0.95

95% confidence interval for (
`p_1-p_2`) =

[
`(\hat p_1-\hat p_2)-1.96 * {\sqrt{(\hat p_1(1-\hat p_1))/(n_1)+(\hat p_2(1-\hat p_2))/(n_2) } }`,
`(\hat p_1-\hat p_2)+1.96 * {\sqrt{(\hat p_1(1-\hat p_1))/(n_1)+(\hat p_2(1-\hat p_2))/(n_2) } }`]

= [
`(0.78-0.60)-1.96 * {\sqrt{(0.78(1-0.78))/(150)+(0.60(1-0.60))/(170) } }` ,
`(0.78-0.60)+1.96 * {\sqrt{(0.78(1-0.78))/(150)+(0.60(1-0.60))/(170) } }` ]

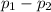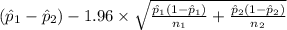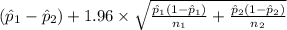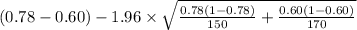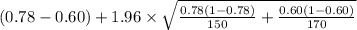= [0.081 , 0.279]

Therefore, 95% confidence interval estimate of the difference between the proportion of women and men who trust recommendations made on LinkedIn is [0.081 , 0.279].