Question

Suppose you have two meter sticks, one made of steel and one made of invar (an alloy of iron and nickel), which are the same length (1.00 m) at 0°C. The coefficients of volume expansion for steel and invar are 3.6 × 10-5 /°C and 2.7 × 10-6 /°C respectively.What is their difference in length, in meters, at 20.5°C ?

Answer 1

• The difference in length for steel is 2.46 x 10⁻⁴ m

• The difference in length for invar is 1.845 x 10⁻⁵ m

Step-by-step explanation:

Given;

original length of steel, L₁ = 1.00 m

original length of invar, L₁ = 1.00 m

coefficients of volume expansion for steel,
`\gamma_(st.)` = 3.6 × 10⁻⁵ /°C

coefficients of volume expansion for invar,
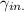`\gamma_(in.)` = 2.7 × 10⁻⁶ /°C

temperature rise in both meter stick, θ = 20.5°C

Difference in length, can be calculated as:

L₂ = L₁ (1 + αθ)

L₂ = L₁ + L₁αθ

L₂ - L₁ = L₁αθ

ΔL = L₁αθ

Where;

ΔL is difference in length

α is linear expansivity =
`(\gamma)/(3)`

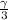
Difference in length, for steel at 20.5°C:

ΔL = L₁αθ

Given;

L₁ = 1.00 m

θ = 20.5°C

`\alpha = (\gamma)/(3) = (3.6*10^(-5))/(3) = 1.2*10^(-5) /^oC`

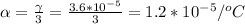
ΔL = 1 x 1.2 x 10⁻⁵ x 20.5 = 2.46 x 10⁻⁴ m

Difference in length, for invar at 20.5°C:

ΔL = L₁αθ

Given;

L₁ = 1.00 m

θ = 20.5°C

`\alpha = (\gamma)/(3) = (2.7*10^(-6))/(3) = 0.9*10^(-6)/^oC`

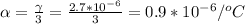
ΔL = 1 x 0.9 x 10⁻⁶ x 20.5 = 1.845 x 10⁻⁵ m