Question

A local medical research association proposes to sponsor a footrace. The average time it takes to run the course is 45.8 minutes with a standard deviation of 3.6 minutes. Assume that the variable is normally distributed. If the association decides to include only the top 20% of the racers, answer the following questions:_________.

a) What should be the cutoff time in the tryout run?
b) Would a person who runs the course in 40 minutes qualify? Justify your answer.

Answer 1

a) The cutoff time in the tryout run is 42.78 minutes.

b) 40 minutes is below 42.78 minutes. So a person who runs the course in 40 minutes qualifies.

Explanation:

Problems of normally distributed samples are solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the zscore of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.

In this problem, we have that:

`\mu = 45.8, \sigma = 3.6`

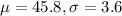
a) What should be the cutoff time in the tryout run?

The top 20% of the racers are the 20% fastest, that is, the 20% that take the less time to run the course.

So the cutoff time is the 20th percentile.

The 20th percentile is X when Z has a pvalue of 0.2, so X when Z = -0.84.

`Z = (X - \mu)/(\sigma)`

`-0.84 = (X - 45.8)/(3.6)`

`X - 45.8 = -0.84*3.6`

`X = 42.78`

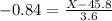
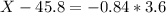
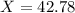
The cutoff time in the tryout run is 42.78 minutes.

b) Would a person who runs the course in 40 minutes qualify? Justify your answer.

The cutoff time in the tryout run is 42.78 minutes. This means that those who ran the course in at most 42.78 minutes qualify.

40 minutes is below 42.78 minutes. So a person who runs the course in 40 minutes qualifies.