Answer:
They bisect each other.
Explanation:
→Solution,
Here, coordinate of quadrilateral DEFG are D(-3,-5), E(-4,3), F(2,6) and G(3,-2).
⇒For diagonal DF.
Let,(x1,y1)=(-3,-5)
(X2,Y2)=(2,6)
→Using midpoint formula.
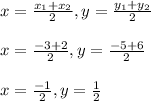
⇒For diagonal EG.
Let,(x1,y1)=(-4,3)
(X2,Y2)=(3,-2)
→Using midpoint formula.
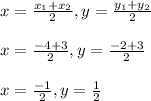
Here, the midpoint of diagonal DF and EG is equal.
Hence they bisect each other.