Answer:
58 children , 29 adults and 76 students attended the theater
Explanation:
Let x be the number of children
We are given that There are half as many adults as there are children.
So, Number of adults =

Let y be the no. of students
A movie theater has a seating capacity of 163.
So,

2x+x+2y=326
3x+2y=326 ----1
The theater charges $5.00 for children, $7.00 for students, and $12.00 of adults.
Cost of x tickets of children = 5x
Cost
 tickets of adults =
tickets of adults =

Cost of y tickets of students = 7y
The total ticket sales was $ 1170
So, 5x+6x+7y=1170
11x+7y=1170 ----2
Plot equation 1 and 2
3x+2y=326 --- Black line
11x+7y=1170 --- red line
Intersection point will give the solution.
Intersection point =(x,y)=(58,76)
Number of children attended = 58
Number of adults attended =
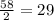
Number of students attended = 76
Hence 58 children , 29 adults and 76 students attended the theater