Question

A supervisor records the repair cost for 17 randomly selected stereos. A sample mean of $66.34 and standard deviation of $15.22 are subsequently computed. Determine the 80% confidence interval for the mean repair cost for the stereos. Assume the population is approximately normal. Step 1 of 2: Find the critical value that should be used in constructing the confidence interval. Round your answer to three decimal places.

Answer 1

Critical value: T = 1.337

The 80% confidence interval for the mean repair cost for the stereos is between $45.991 and $86.689

Explanation:

We have the standard deviation for the sample. So we use the t-distribution to solve this question.

The first step to solve this problem is finding how many degrees of freedom, we have. This is the sample size subtracted by 1. So

df = 17 - 1 = 16

80% confidence interval

Now, we have to find a value of T, which is found looking at the t table, with 16 degrees of freedom(y-axis) and a confidence level of
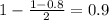`1 - (1 - 0.8)/(2) = 0.9`. So we have T = 1.337, which is the critical value

The margin of error is:

M = T*s = 1.337*15.22 = 20.349

In which s is the standard deviation of the sample.

The lower end of the interval is the sample mean subtracted by M. So it is 66.34 - 20.349 = $45.991

The upper end of the interval is the sample mean added to M. So it is 66.34 + 20.349 = $86.689

The 80% confidence interval for the mean repair cost for the stereos is between $45.991 and $86.689