Question

For what values of h are the vectors [Start 3 By 1 Matrix 1st Row 1st Column negative 1 2nd Row 1st Column 4 3rd Row 1st Column 6 EndMatrix ]​, [Start 3 By 1 Matrix 1st Row 1st Column 5 2nd Row 1st Column 2 3rd Row 1st Column negative 3 EndMatrix ]​, [Start 3 By 1 Matrix 1st Row 1st Column 3 2nd Row 1st Column negative 5 3rd Row 1st Column negative 4 EndMatrix ]​, and [Start 3 By 1 Matrix 1st Row 1st Column 12 2nd Row 1st Column negative 20 3rd Row 1st Column h EndMatrix ]linearly​ dependent?

Answer 1

The set is linearly dependent for every value of h.

Explanation:

Recall that a set of vector {a,b,c,d} is a linearly dependent set of vectors if any of the vectors can be written as a linear combination of the other ones.

We are given the following vectors.a=(-1,4,6), b=(5,2,-3), c=(3,-5,-4), d=(12,-20, h). At first, we will check if a,b,c are linearly independent. To do so, we will calculate the following determinant (the procedure of the calculation is omitted).

`\left|\begin{matrix}-1 & 5 & 3 \\ 4 & 2 & -5 & \\ 6 & -3 & -4\end{matrix}\right| = -119\\eq 0`

Since the determinant is not zero, this implies that the vectors a,b,c are all linearly independent. Since a,b,c are all vectors in
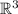`\mathbb{R}^3` which is a 3-dimensional space, and they are 3 linear independent vectors, then they are automatically a base of this space. Consider now the vector d. Since {a,b,c} is a base of
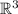`\mathbb{R}^3`, then it generates any vector of this space(i.e any other vector of the space is a linear combination of {a,b,c}). In particular, d. So the set {a,b,c,d} is linearly independent for any value of h