Answer:
the solution is in the explanation
Step-by-step explanation:
q = D(p) = 0.25(250 -
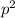 )
)
 /
/
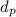 = 0.25 (0-2p) = -0.5p
= 0.25 (0-2p) = -0.5p
a. Elasticity E(p) =
![\left[\begin{array}{ccc}p/q\\:\\d_(q)/d_(p) \end{array}\right]](https://img.qammunity.org/2021/formulas/business/college/f5emvndt56k7x3kbnbfrpr0jvixhwx540j.png)
E(p) =
![\left[\begin{array}{ccc}p/0.25(270-p^(2)) X (-0.5)P \end{array}\right]](https://img.qammunity.org/2021/formulas/business/college/w8ori0r5s1h3z5avh1y3et8dt2f9uti06k.png)
E(P) = 2
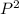 /270-
/270-
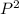
b. E(10) =2(100)/ 270 - 100 = 200/170 = 1.18
c. E(10) > 1
so the demand is elastic
hence unit price be lowered to increase revenue
yes
d. for maximum revenue: E(p) = 1
2
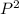 /270-
/270-
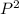 = 1
= 1
cross multiply you have 3
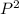 = 270
= 270
P =
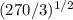
P = 9.48 dollars