Answer:
a) 2.34x
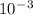 N
N
b) 7.91 x
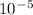 C
C
Step-by-step explanation:
a)The centripetal force is given by
F= mv²/r
For radius, using the equation i.e s=
 (2πr) and substituting s=vt there gives,
(2πr) and substituting s=vt there gives,
r=
 =>
=>
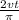
Now, using the equation for magnitude of magnetic force
F= mv²/r => mv²/
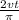
F= [(3.142)(6.81 x
 kg)(62m/s)] / (2)(2.83x
kg)(62m/s)] / (2)(2.83x
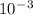 s)
s)
F= 2.34x
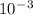 N
N
b) In order to find the magnitude |q| of the charge, we'll use the equation
F= |q|vBsinθ
|q|= F/vBsin(90°) => 2.34x
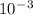 /(62 x 0.477 x 1)
/(62 x 0.477 x 1)
|q|= 7.91 x
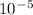 C
C