Question

One hundred turns of insulated copper wire are wrapped into a circular coil of crosssectional area 1.20⇥103 m2. The two ends of the wire are connected to an ammeter. The total resistance in the circuit is 1.30 ⌦. If an externally applied uniform magnetic field perpendicular to the plane of the coil changes from 1.60 T in one direction to 1.60 T in the opposite direction in 1.5 seconds, what is the current through the ammeter? Hint: Start with Faraday’s Law.

Answer 1

236.3 x
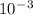`10^-^3` C

Step-by-step explanation:

Given:

B(0)=1.60T and B(t)=-1.60T

No. of turns 'N' =100

cross-sectional area 'A'= 1.2 x
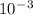`10^-^3`m²

Resistance 'R'= 1.3Ω

According to Faraday's law, the induced emf is given by,

ℰ=-NdΦ/dt

The current given by resistance and induced emf as

I = ℰ/R

I= -NdΦ/dtR

By converting the current to differential form(the time derivative of charge), we get

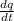`(dq)/(dt)`= -NdΦ/dtR

dq= -N dΦ/R

The change in the flux dФ =Ф(t)-Ф(0)

therefore, dq =
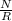`(N)/(R)` (Ф(0)-Ф(t))

Also, flux is equal to the magnetic field multiplied with the area of the coil

dq = NA(B(0)-B(t))/R

dq= (100)(1.2 x
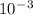`10^-^3`)(1.6+1.6)/1.3

dq= 236.3 x
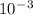`10^-^3` C