Question

A blood test indicates the presence of a particular disease 95% of the time when the disease is actually present. The same test indicates the presence of the disease 0.5% of the time when the disease is not actu- ally present. One percent of the population actually has the disease. Calculate the probability that a person actually has the disease given that the test indicates the presence of the disease.

Answer 1

Probability that a person actually has the disease given that the test indicates the presence of the disease is 0.657.

Explanation:

We are given that a blood test indicates the presence of a particular disease 95% of the time when the disease is actually present.

The same test indicates the presence of the disease 0.5% of the time when the disease is not actually present. One percent of the population actually has the disease.

Let the Probability that person actually has the disease = P(A) = 0.01

Probability that person actually doesn't has the disease = P(A') = 1 - P(A) = 1 - 0.01 = 0.99

Also, let PD = event that there is a presence of the disease

So, Probability that test indicates the presence of the disease given the fact that the disease is actually present = P(PD/A) = 0.95

Probability that test indicates the presence of the disease given the fact that the disease is not actually present = P(PD/A') = 0.005

Now, the probability that a person actually has the disease given that the test indicates the presence of the disease = P(A/PD)

We will use Bayes' theorem to calculate the above probability.

SO, P(A/PD) =
`(P(A) * P(PD/A))/(P(A) * P(PD/A) + P(A')* P(PD/A'))`

=
`(0.01 * 0.95)/(0.01 * 0.95+ 0.99* 0.005)`

=
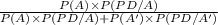
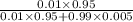
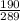`(190)/(289)` = 0.657

Hence, the required probability is 0.657.