Question

An environmentalist group collects a liter of water from each of 52 random locations along a stream and measures the amount of dissolved oxygen in each specimen. The mean is 2.78 milligrams (mg). Is this strong evidence that the stream has a mean oxygen content of less than 3 mg per liter? (Suppose we know that dissolved oxygen varies among locations according to a Normal distributions with σ = 0.85 mg. Use α = 0.05.)

Answer 1

`z=(2.78-3)/((0.85)/(√(52)))=-1.866`

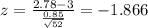
Now we can calculate the p value given by:

`p_v =P(z<-1.866)=0.031`

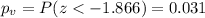
Since the p value is lower than the significance level of 0.05 given we have enough evidence to REJECT the null hypothesis and the conclusion would be that the true mean for the oxygen content is ignificantly less than 3 mg per liter

Explanation:

Info given

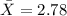`\bar X=2.78` represent the mean for the amount of dissolved oxygen

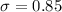`\sigma=0.85` represent the population standard deviation

`n=52` sample size selected

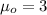`\mu_o =3` represent the level that we want to verify

`\alpha=0.05` represent the significance level

z would represent the statistic

`p_v` represent the p value

System of hypothesis

In the problem we want to determine if the stream has a mean oxygen content of less than 3 mg per liter, the hypothesi are:

Null hypothesis:
`\mu \geq 3`

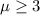
Alternative hypothesis:
`\mu < 3`

Since we know the population deviation the statistic can be calculated like this:

`z=(\bar X-\mu_o)/((s)/(√(n)))` (1)

Replacing the info given we got:

`z=(2.78-3)/((0.85)/(√(52)))=-1.866`

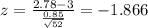
Now we can calculate the p value given by:

`p_v =P(z<-1.866)=0.031`

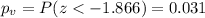
Since the p value is lower than the significance level of 0.05 given we have enough evidence to REJECT the null hypothesis and the conclusion would be that the true mean for the oxygen content is ignificantly less than 3 mg per liter