Question

The connection is made using a bolt and nut and two washers. If the allowable bearing stress of the washers on the boards is (sb)allow = 2 ksi, and the allowable tensile stress within the bolt shank S is (st)allow = 18 ksi, determine the maximum allowable tension in the bolt shank. The bolt shank has a diameter of 0.31 in., and the washers have an outer diameter of 0.75 in. and inner diameter (hole) of 0.50 in.

Answer 1

P = 0.490 kip

Step-by-step explanation:

given data

allowable bearing stress = 2 ksi

allowable tensile stress = 18 ksi

diameter = 0.31 in

outer diameter = 0.75 in

inner diameter (hole) = 0.50 in

solution

we find here cross section area of shank that is express as

Area =
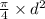`(\pi )/(4) * d^2` ..................1

area =
`(\pi )/(4) * 0.31^2`

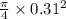
area = 0.0754 in²

and

now we get here allowable load in bolt will be

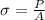`\sigma = (P)/(A)` ...................2

P =
`\sigma * A`

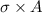
P = 18 × 10³ × 0.0754

P = 1357.2 = 1.357 kip

and

now find here area of washer is

Area =
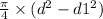`(\pi )/(4) * (d^2-d1^2)` .......................3

put here value

Area =
`(\pi )/(4) * (0.75^2-0.5^2)`

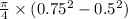
area = 0.2454 in²

so now we get here allowable load of washer will be

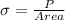`\sigma = (P)/(Area)` .....................4

P = 2 × 10³ × 0.245

P = 490 = 0.490 kip