Question

Paul owns a mobile wood-fired pizza oven operation. A couple of his clients complained about his dough at a recent catering, so he changed his dough to a newer product. Using the old dough, there were 6 complaints out of 385 pizzas. With the new dough, there were 16 complaints out of 340 pizzas. Let p1 be the proportion of customer complaints with the old dough and p2 be the proportion of customer complains with the new dough. Based on a 95% confidence for the difference of the proportions, what can be concluded?

Answer 1

`(0.016-0.047) - 1.96 \sqrt{(0.016(1-0.016))/(385) +(0.047(1-0.047))/(340)}=-0.057`

`(0.016-0.047) + 1.96 \sqrt{(0.016(1-0.016))/(385) +(0.047(1-0.047))/(340)}=-0.0052`

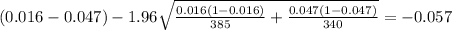
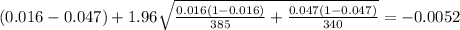
And the confidence interval for the true difference of proportions is given by:

`-0.057 \leq p_1 -\hat p_2 \leq -0.0052`

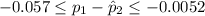
Since the confidence interval not contains the value 0 and all the values are negative we have enough evidence to conclude that the proportion for the old dough is significantly less than the new dough

Explanation:

We have the information given:

`p_1` represent the real population proportion of complaints for the old dough

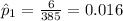`\hat p_1 =(6)/(385)=0.016` represent the estimated proportion of complaints for the old dough

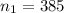`n_1=385` is the sample size for the old dough

`p_2` represent the real population proportion of complaints for the new dough

`\hat p_2 =(16)/(340)=0.047` represent the estimated proportion of complaints for the new dough

`n_2=340` is the sample size for the new dough

`z` represent the critical value

The confidence interval for the difference of two proportions would be given by this formula :

`(\hat p_1 -\hat p_1) \pm z_(\alpha/2) \sqrt{(\hat p_1(1-\hat p_1))/(n_1) +(\hat p_2 (1-\hat p_2))/(n_2)}`

The confidence level is 95% the significance level is
`\alpha=1-0.95=0.05` and
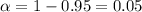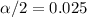`\alpha/2=0.025`, and the critical value for this case would be.

`z_(\alpha/2)=1.96`

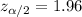
Replacing the info given into the confidence interval we got:

`(0.016-0.047) - 1.96 \sqrt{(0.016(1-0.016))/(385) +(0.047(1-0.047))/(340)}=-0.057`

`(0.016-0.047) + 1.96 \sqrt{(0.016(1-0.016))/(385) +(0.047(1-0.047))/(340)}=-0.0052`

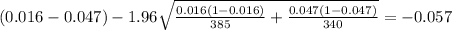
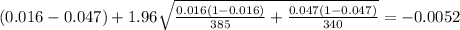
And the confidence interval for the true difference of proportions is given by:

`-0.057 \leq p_1 -\hat p_2 \leq -0.0052`

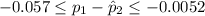
Since the confidence interval not contains the value 0 we have enough evidence to conclude that the proportion for the old dough is significantly less than the new dough