Answer:
 , assuming that the two cylinders are of the same height.
, assuming that the two cylinders are of the same height.
Explanation:
The volume of a cylinder of radius
 and height
and height
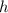 is
is
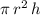 .
.
If the two cylinders are of the same height
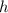 , the volume of the two cylinders would be proportional to the square of their radii.
, the volume of the two cylinders would be proportional to the square of their radii.
Let
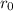 and
and
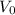 denote the radius and volume of the smaller cylinder. Similarly, let
denote the radius and volume of the smaller cylinder. Similarly, let
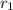 and
and
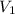 denote the radius and volume of the larger cylinder. Assuming that the height of both cylinders is
denote the radius and volume of the larger cylinder. Assuming that the height of both cylinders is
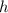 :
:
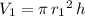 .
.
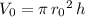 .
.
Hence,
 (ratio between the volume of the larger cylinder and the volume of the smaller cylinder) would be:
(ratio between the volume of the larger cylinder and the volume of the smaller cylinder) would be:
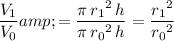 .
.
In this question, it is given that:
- the volume of the smaller cylinder is
 .
. - the radius of the smaller cylinder is
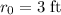 .
. - the radius of the larger cylinder is
 .
.
Rearrange the equation
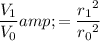 to find an expression for
to find an expression for
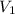 , volume of the larger cylinder:
, volume of the larger cylinder:
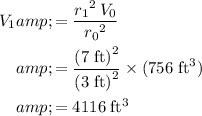 .
.