Answer:
0.61
Explanation:
The computation of the probability of the working of the system is shown below:
But before that first we have to find out the total probability of sub-system i.e 2,3 and 4 i.e
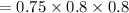
= 0.48
Now the working of the system probability is
= 1 - [(1 - probability of component 1) × (1 - sub system probability)]
= 1 - [(1 - 0.75) × (1 - 0.48)]
= 1 - 0.39
= 0.61
Please find the attachment for better understanding.