Question

If 3x − y = 12, what is the value of 8^x/2^y?

A) 2^12
B) 4^4
C) 8^2
D) The value cannot be determined from the information given.

Answer 1

A

Step-by-step explanation:

Let's solve the equation to be written as y in terms of x by adding y to and subtracting 12 from both sides:

3x - y = 12

3x - 12 = y

Now, replace y in the other expression that we want to find (
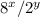`8^x/2^y`) with this expression 3x - 12:

`8^x/2^y`

`8^x/2^(3x-12)`

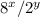
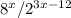
Now, we also know that 8 = 2³, so substitute 2³ for 8 in the expression:

`8^x/2^(3x-12)`

`(2^3)^x/2^(3x-12)=2^(3x)/2^(3x-12)`

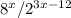
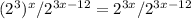
Remember that when dividing powers with the same base (in this case, the common base is 2), the result will be a single power whose exponent is the difference of the other two powers. Here, then we have:

`2^(3x)/2^(3x-12)=2^(3x-(3x-12))=2^(12)`

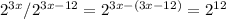
Thus, the answer is A.