How do you differentiate y=x^(e^x) ?

Question

asked Apr 1, 2023 48.2k views

1 Answer

Zhijian Lin · Answer 1 · 2023-04-07T06:25:55+0000

We are given the function to differentiate:

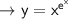
${\quad \qquad \sf \rightarrow y=x^(e^x)}$

Do take natural log on both sides, then we will be having

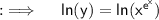
${:\implies \quad \sf ln(y)=ln(x^{e^(x)})}$

${:\implies \quad \sf ln(y)=e^(x)ln(x)\quad \qquad \{\because ln(a^b)=bln(a)\}}$

Now, differentiate both sides by using so called chain rule and the product rule

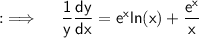
${:\implies \quad \sf (1)/(y)(dy)/(dx)=e^(x)ln(x)+(e^x)/(x)}$

${:\implies \quad \boxed{\bf{(dy)/(dx)=x^(e^x)\bigg\{(e^x)/(x)+ln(x)e^(x)\bigg\}}}}$

Hence, Option B) is correct

Product rule of differentiation:

Where, u and v are functions of x