Question

A company produces batteries. On Average, 85% of all batteries are produced are good.Each battery is tested before being dispacted, and the inspector correctly classifies the battery 90% of the time.

A. What percentage of the batteries will "classified as good"?
B.What is the probability that a battery is defective given that it was classified as good?​

Answer 1

a) 78% of the batteries will be classified as good.

b) 1.92% probability that a battery is defective given that it was classified as good

Explanation:

For question b, the conditional probability formula will be used.

Conditional probability formula:

`P(B|A) = (P(A \cap B))/(P(A))`

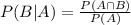
In which

P(B|A) is the probability of event B happening, given that A happened.

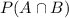`P(A \cap B)` is the probability of both A and B happening.

P(A) is the probability of A happening.

A. What percentage of the batteries will "classified as good"?

85% of the batteries are good. The inspector correctly classifies the battery 90% of the time, which means that of those 90% will be classified as good.

100-85 = 15% of the batteries are not good. Of those, 100-90 = 10% will be classified as good. Then

0.85*0.9 + 0.15*0.1 = 0.78

78% of the batteries will be classified as good.

B.What is the probability that a battery is defective given that it was classified as good?​

Event A: classified as good.

Event B: Defective

From A, P(A) = 0.78

Intersection:

100-85 = 15% of the batteries are not good. Of those, 100-90 = 10% will be classified as good.

This means that
`P(A \cap B) = 0.15*0.1 = 0.015`

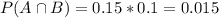
Then

`P(B|A) = (P(A \cap B))/(P(A)) = (0.015)/(0.78) = 0.0192`

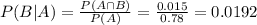
1.92% probability that a battery is defective given that it was classified as good