Question

A set of art exam scores are normally distributed with a mean of 81 points and a standard deviation of 10

points. Kamil got a score of 78 points on the exam.
What proportion of exam scores are lower than Kamil's score?
You may round your answer to four decimal places

Answer 1

is 0.3821

Explanation:

just answered it on khan

Answer 2

We have been given that a set of art exam scores are normally distributed with a mean of 81 points and a standard deviation of 10 points. Kamil got a score of 78 points on the exam. We are asked to find the proportion of exam scores that are lower than Kamil's score.

First of all, we will find z-score corresponding to 78.

`z=(x-\mu)/(\sigma)`, where,

z = z-score,

x = Random sample score,

`\mu` = Mean,

`\sigma` = Standard deviation.

`z=(78-81)/(10)`

`z=(-3)/(10)`

`z=-0.3`

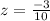
Now we will use normal distribution table to find the probability under a z-score of
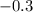`-0.3` that is
`P(z<-0.3)`.

`P(z<-0.3)=0.38209`

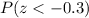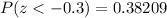
Upon rounding to 4 decimal places, we will get:

`P(z<-0.3)\approx 0.3821`

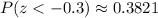
Therefore,
`0.3821` of exam scores are lower than Kamil's score.