Question

In T-ball, the distance to each successive base is 50 feet. If the distance from home plate to the pitcher’s mound is 38 feet, how far is it from the pitcher’s mound to 2nd base? Show this distance is approximately 32.7 feet using two different methods.

Answer 1

The distance from the pitcher's mound and to second base is 37.99 approximately.

Explanation:

The diamond is a square, which in this case has 50 feet long each side, and from home to pitcher is 38 feet. Notice that home is a vertex of the square and the pitcher's mound is the intersection of the diagonals, where they cut half.

We can find the distance from the pitcher to first base using Pythagorean's Theorem, where 50 feet is the hypothenuse.

`50^(2) =38^(2)+x^(2)\\x^(2)=50^(2)-38^(2)\\x=√(2500-1444)\\ x=√(1056)\\ x \approx 32.5 \ ft`

Therefore, the distance from the pitcher to first base is 32.5 feet, approximately.

Now, we can use again Pythagorean's Theorem to find the distance from pitcher to second base, where the hypothenuse is 50 feet.

`50^(2)=32.5^(2)+y^(2)\\y^(2)=50^(2)-32.5^(2)\\y=√(2500-1056.25)\\ y =√(1443) \approx 37.99`

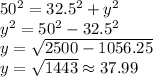
Therefore, the distance from the pitcher's mound and to second base is 37.99 approximately.

(this results make sense, because the diagonals of a square intersect at half, that means all bases have the same distance from pitcher's mound, so the second way to find the distance asked in the question is just using theory)