Question

The perimeter of a rectangle is 40cm. If the length were doubled and the width halved, the perimeter would be increased by 16cm. Find the dimensions of the original rectangle.

Create a linear system to model the situation.
Determine, by substitution, the dimensions of the original rectangle (sketch and label rectangles to help) PLS SHOW ALL WORK NEED ASAP

Answer 1

The dimensions of the original rectangle are Length =12cm, Width=8cm

Explanation:

Let the length of the rectangle be x

Let the width of the rectangle be y

The perimeter of a rectangle is 40cm.

2(x+y)=40

Divide both sides by 2

x+y=20

If the length were doubled(2x) and the width halved(y/2), the perimeter would be increased by 16cm,i.e.(40+16)cm

Therefore:

`2(2x+(y)/(2))=56`

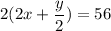
Divide both sides by 2

`2x+(y)/(2)=28`

From the first equation, x=20-y.

Substitute x=20-y into
`2x+(y)/(2)=28`

`2x+(y)/(2)=28`

`2(20-y)+(y)/(2)=28\\(4(20-y)+y)/(2)=28\\80-4y+y=56\\-3y=-24\\$Divide both sides by -3\\y=8cm\\Recall:x=20-y\\x=20-8=12cm\\The dimensions of the original rectangle are Length =12cm, Width=8cm`