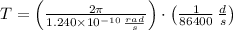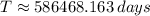Answer:
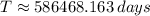
Step-by-step explanation:
The initial angular speed of the Sun is:

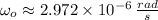
Let suppose that Sun can be modelled as an uniform sphere, the moment of inertia is:

The initial moment of inertia is:
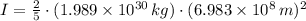
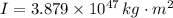
The angular momentum is:
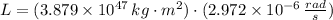
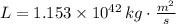
Given the absence of external forces exerted on the Sun, the final angular speed can found by the Principle of Angular Momentum Conservation. The final moment of inertia is:
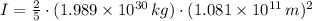
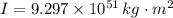
The final angular speed is:


The period of rotation is: