Question

How does the volume of a cylinder with a radius of 3 units and a height of 12 units compare to the volume of a rectangular prism with dimensions 16 units x 16 units x 9 units?

a. The volume of the cylinder is greater than the the volume of the prism

b. The volume of the cylinder is smaller than the volume of the prism.

C. The volume of the cylinder is the same as the volume of the prism

d.You cannot compare the volumes of different shapes

Answer 1

B: The volume of the cylinder is smaller than the volume of the prism.

Explanation:

In order to find the volume of the cylinder, we would use to formula
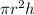`\pi r^(2) h`. Substitute the given measures of the cylinder to get
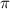`\pi`·3²·12. The simplified and calculated form would be 108 units cubed.

Now, we find the volume of the rectangular prism, which is
`l`·
`w`·
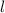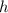`h`, or 16*16*9. This means the volume is 2304 units cubed.

Therefore, since 2304 un³>108 un³, answer choice b would be correct.

Answer 2

The volume of the cylinder is greater than the volume of the prism.

Explanation:

The Volume of a cylinder is given as:

= πr²h

Therefore, the volume of a cylinder with a radius of 3 units and a height of 12 units will be:

= πr²h
=3.14 × 3² × 12

=339.12

The volume of a rectangular prism with dimensions 3 units x 3 units x 12 units will be:

= Length × Width × Height

Based on the calculation, the volume of the cylinder is greater than the volume of the prism.