Question

Vignesh owns a cottage in the shape of a cube with each edge of length 26 feet. The roof is in the shape of a square pyramid and it extends two feet over the edge of the cottage on each side. The lateral sides of the roof are 17 feet long. What is the total surface area of the roof?

Answer 1

1,244 ft²

Explanation:

Lateral surface area of the roof:

4(½ × (26+4) × 17)

1020

Part of the base:

(26+4)² - 26²

30² - 26²

224

Total surface area:

1020 + 224

1244

Answer 2

56√93 ≈ 540 ft²

Explanation:

The roof is made up of 4 congruent isosceles triangles. Since the roof extends 2 ft over the edge of the cottage on each side, then the base of each triangle is 26 + 2 = 28 ft.

Let's calculate the area of one of the triangles and then just multiply by 4. See attachment. Drop a perpendicular from the vertex of a triangle to its base. We now have the triangle broken up into two right triangles. The hypotenuse is 17 ft and one of the legs of the right triangle is 28 / 2 = 14 ft. Then the height / other leg is:

`√(17^2-14^2) =√(289-196) =√(93)`

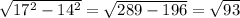
We now have the dimensions of each triangle: the height is √93 and the base is 28. The area of a triangle is: A = (1/2) * b * h, so we have the following.

A = (1/2) * b * h

A = (1/2) * 28 * √93 = 14√93

Multiply this by 4:

14√93 * 4 = 56√93

The total surface area is 56√93 ≈ 540 ft².