Question

Suppose point z is halfway between point w and x a standard numberline, and point x is halfway between points z and y. Where is w if z is located at 1/3and y is located at 11/3

Answer 1

Answer: d

Explanation:

Answer 2

The point w is located at -4/3

Explanation:

Point z is halfway between point w and x

Point x is halfway between point z and y

Point z is located at 1/3 and point y is located at 11/3

Please refer to the number line attached

Then the x is located at the mid-point of z and y

`x = (z+y)/(2) \\\\x = ((1)/(3)+ (11)/(3) )/(2)`

`x = 2`

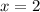
Since z is the mid-point of w and x

`z = (w+ x )/(2)\\\\(1)/(3) = (w+ 2 )/(2)\\\\(2)/(3) = w+ 2 \\\\w = (2)/(3) - 2\\\\w = -(4)/(3)`

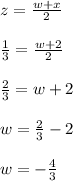
Therefore, the point w is located at -4/3