Question

It is advertised that the average braking distance for a small car traveling at 65 miles per hour is less than 120 feet. A transportation researcher wants to determine if the statement made in the advertisement is false. She randomly test drives 36 small cars at 65 miles per hour and records the braking distance. The sample average braking distance is computed as 115 feet. Assume that the population standard deviation is 22 feet.

1) State the null and the alternative hypotheses for the test

2) Calculate the value of the test statistic and the p-value.

3) Use to determine if the average breaking distance differs from 120 feet

4) Repeat the test using the critical value approach.

Answer 1

a) Null hypothesis:
`\mu \geq 120`

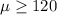
Alternative hypothesis:
`\mu < 120`

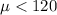
b)
`z=(115-120)/((22)/(√(36)))=-1.36`

`p_v =P(Z<-1.36)=0.0869`

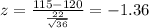
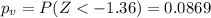
c)We don't have a significance level provided but at 1% of 5% of significance we can conclude that the true mean for the breaking distance is not significantly different from 120 since the p value is higher than the significance levels assumed.

d) At 5% of significance the critical value is
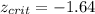`z_(crit)= -1.64` and since the calculated statistic is not lower than the critical value we don't have enough evidence to conclude that the true mean is significanylt less than 120 ft

At 1% of significance the critical value is
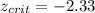`z_(crit)= -2.33` and since the calculated statistic is not lower than the critical value we don't have enough evidence to conclude that the true mean is significanylt less than 120 ft

Explanation:

Information given by the problem

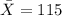`\bar X=115` represent the sample mean for the breaking distance

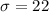`\sigma=22` represent the sample population deviation

`n=36` sample size selected

`\mu_o =120` represent the value to verify

t would represent the statistic

`p_v` represent the p value

Part a

We want to conduct a test in order to see if the average braking distance for a small car traveling at 65 miles per hour is less than 120 feet, the system of hypothesis would be:

Null hypothesis:
`\mu \geq 120`

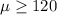
Alternative hypothesis:
`\mu < 120`

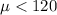
Part b

Since we know the population deviation we can calculate the statistic like this:

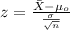`z=(\bar X-\mu_o)/((\sigma)/(√(n)))` (1)

Replacing the data given we got:

`z=(115-120)/((22)/(√(36)))=-1.36`

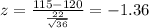
Now we can calculate the p value, we have a left tailed test then p value would be:

`p_v =P(Z<-1.36)=0.0869`

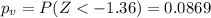
Part c

We don't have a significance level provided but at 1% of 5% of significance we can conclude that the true mean for the breaking distance is not significantly different from 120 since the p value is higher than the significance levels assumed.

Part d

For this case we need to find a critical value in the normal standard distribution who accumulates the significance level
`\alpha` in the left of the distribution.

At 5% of significance the critical value is
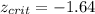`z_(crit)= -1.64` and since the calculated statistic is not lower than the critical value we don't have enough evidence to conclude that the true mean is significanylt less than 120 ft

At 1% of significance the critical value is
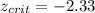`z_(crit)= -2.33` and since the calculated statistic is not lower than the critical value we don't have enough evidence to conclude that the true mean is significanylt less than 120 ft