Question

An electronics firm produces 45,000 calculators annually, and each calculator requires 2 nickel-cadmium batteries (so they use 90,000 batteries per year). The batteries are purchased from a local supplier for $0.14 each, and the supplier charges $250 each time a delivery is made. The annual storage cost is $0.046 per battery, and the cost of capital (i.e., opportunity cost) is 10% per year.

A. What is the annual holding cost per battery (incliding storage cost and cost of capital)?
B. What is the annual inventory cost associated with the batteries if the firm orders 15,000 batteries at a time?
C. What is the economic order quantity?
A. 17,503.
B. 20,194.
C. 27,386.
D. 30,000.

Answer 1

A.

$0.05

B.

$18,600

C.

30,000 units

Step-by-step explanation:

Economic order quantity is the quantity at which business incur minimum cost. This is the level of order where the holding cost equals to the ordering cost of the business.

As per given data

Annual Demand = 90,000 batteries

Ordering cost = $250

Carrying cost = $0.046

A.

Annual Holding cost = Holding cost per unit x Annual Demand = $0.046 x 90,000 batteries = $4,140

Opportunity cost = $0.046 X 110% = $0.05

B.

Purchase cost = 90,000 x $0.14 = $12,600

Ordering cost = (90,000/15,000) x $250 = $1,500

Storage cost = $0.05 x 90,000 = $4,500

Total cost of Inventory = $12,600 + $1,500 + $4,500 = $18,600

C.

EOQ =
`\sqrt{(2 X S X D)/(H) }`

EOQ =
`\sqrt{(2 X 250 X 90000)/(0.05) }`

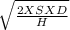
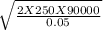
EOQ = 30,000