Question

A string, 30 cm long and having a mass of 45 g, is attached to a 810 Hz oscillator at one end. The other end of the string is fixed and the string is kept under tension. The oscillator produces a transverse wave in the string, whose amplitude is 7.0 m, and which propagates with a velocity of 76 m/s. The energy of the wave is absorbed at the fixed end. In this situation, the tension in the string, in SI units, is closest to:_______.

a. 940.
b. 900.
c. 970.
d. 870.
e. 830.

Answer 1

870 N

Step-by-step explanation:

The expression for velocity of wave in a string is given below

v =
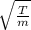`\sqrt{(T)/(m) }` , T is tension and m is mass per unit length .

m = 45 x 10⁻³ / 30 x 10⁻²

= .15 kg/m

Putting the given values in the equation

76 =
`\sqrt{(T)/(.15) }`

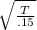
T = 76² x .15

= 866.4

870 N approx.