Question

Performing a study on the development of body height, a student randomly measures the height of 30 persons in country A. The results turn out to be: {1.7, 1.6, 1.8, 1.9, 1.75, 1.83, 1.82, 1.65, 1.95, 1.69, 1.82, 1.87, 1.65, 1.54, 1.98, 1.78, 1.69, 1.75, 1.62, 1.64, 1.75, 1.8, 1.65, 1.7, 1.82, 1.62, 1.83, 1.75, 1.7, 1.65}. In the literature, a result is found that 10 years before, the average height of persons in country A was determined to be 1.73 and standard deviation is 0.2 .

Given that the acceptable threshold for significance is 5%, can this data be used to show that the average height of individuals in country A has increased in the last 10 years?
(Show your calculations).

Answer 1

`t= (1.743-1.73)/((0.107)/(√(30)))= 0.665`

`df = n-1= 30-1=29`

`p_v= P(t_(29)>1.73)= 0.047`

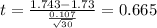
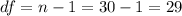
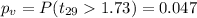
And if we compare the p value obtained and the significance level provided of 0.05 or 5% we see that
`p_v<\alpha` so then we have enough evidence to conclude that the true mean for this case is significantly higher tha 1.73 since we reject the null hypothesis for the test

Explanation:

We have the following dataset given:

1.7, 1.6, 1.8, 1.9, 1.75, 1.83, 1.82, 1.65, 1.95, 1.69, 1.82, 1.87, 1.65, 1.54, 1.98, 1.78, 1.69, 1.75, 1.62, 1.64, 1.75, 1.8, 1.65, 1.7, 1.82, 1.62, 1.83, 1.75, 1.7, 1.65

We can calculate the sample mean and deviation from this data with the following formulas:

`\bar X =(\sum_(i=1)^n X_i)/(n)`

`s =\sqrt{(\sum_(i=1)^n (X_i -\bar X)^2)/(n-1)}`

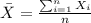
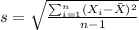
And replacing we got:

`\bar X= 1.743 ,s=0.107 , n=30`

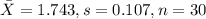
From previous info we know that 10 years before, the average height of persons in country A was determined to be 1.73 and standard deviation is 0.2.

We can use a one t test in order to check the claim that he average height of individuals in country A has increased in the last 10 years

Null hypothesis:
`\mu \leq 1.73`

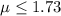
Alternative hypothesis:
`\mu>1.73`

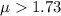
The statistic is given by:

`t=(\bar X \mu)/((s)/(√(n)))`

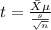
Replacing the info given we got:

`t= (1.743-1.73)/((0.107)/(√(30)))= 0.665`

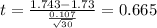
The degrees of freedom for this test are given by:

`df = n-1= 30-1=29`

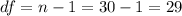
Now we can calculate the p value with the following probability:

`p_v= P(t_(29)>1.73)= 0.047`

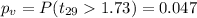
And if we compare the p value obtained and the significance level provided of 0.05 or 5% we see that
`p_v<\alpha` so then we have enough evidence to conclude that the true mean for this case is significantly higher tha 1.73 since we reject the null hypothesis for the test