Answer:
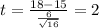
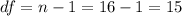
And the p value since we have a right tailed test is given by:
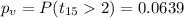
And for this case the p value is higher than the significance level of 0.05 so then we have enough evidence to FAIL to reject the null hypothesis and the best conclusion for this case is:
t = 2, p-value > 5%, fail to reject the null hypothesis. There is not enough evidence to conclude that the mean drying time is greater than 15 minutes.
Explanation:
For this problem we have the following info given:
 represent the sample size
represent the sample size
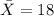 represent the sample mean for the drying time
represent the sample mean for the drying time
 represent the sample deviation
represent the sample deviation
We want to test the claim that the mean drying time of their product is, at most, 15 minutes, so then the system of hypothesis are:
Null hypothesis:
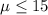
Alternative hypothesis:
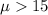
The statistic is given by this formula since we don't know the population deviation:
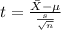
And replacing we have:
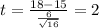
Now we can find the degrees of freedom given by:
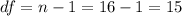
And the p value since we have a right tailed test is given by:
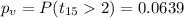
And for this case the p value is higher than the significance level of 0.05 so then we have enough evidence to FAIL to reject the null hypothesis and the best conclusion for this case is:
t = 2, p-value > 5%, fail to reject the null hypothesis. There is not enough evidence to conclude that the mean drying time is greater than 15 minutes.