Answer:
(-1, -1)
Explanation:
Given equation of the perpendicular transversal:
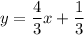
To find which ordered pair would be on the perpendicular transversal, simply input each value of x into the equation:
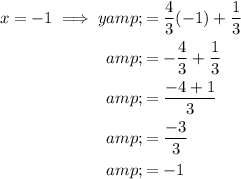
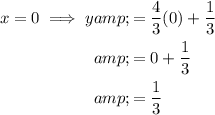
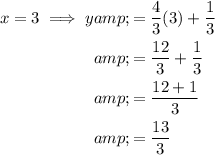
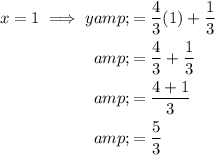
Therefore, the only ordered pair that is on the perpendicular transversal is: