Answer:
(a)v(t)=38t-10
(b)
Explanation:
Given the position of a function, s(t) at time t as:
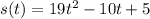
(a)Velocity, v(t)
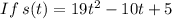
Then:
s'(t)=38t-10
The velocity, v(t) of the function at time (t) is:
v(t)=38t-10
(b)If v(t)=38t-10
At t=0, v(0)=38(0)-10=-10
At t=2, v(2)=38(2)-10=66
At t=9, v(9)=38(9)-10=332
We are not given units, but velocity is always a function of distance per time.