Answer: 1. It is not possible to construct a triangle of given measure sides.
2. The value of ∠A is 44°
Explanation:
1.
Given: Three sides of a triangle
Let a= 12 m , b=30 m and c= 18 m
Now as we know
The sum of two sides of a triangle is always greater than the third side
Therefore we need to show
a+b>c -----(i)
b+c>a -----(ii)
c+a>b -----(iii)
(i a+b= 12+30 = 42>18= b ⇒ a+b>c
ii) b+c=30+18= 48 >12=a ⇒ b+c>a
iii) c+a=18+12=30=b ⇒ c+a= b
Therefore the third condition does not verified
Hence, it is not possible to construct a triangle of given measure sides.
2.
As we now triangle sum property which states that the sum of all the three angles of a triangle is 180°
So we have
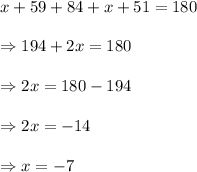
Therefore
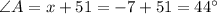
Hence, the value of ∠A is 44°