Answer:
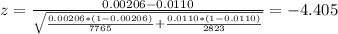
Now we can find the p value since we are using a left tailed test the p value would be:
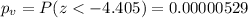
Since the p value is very low compared to the significance level of 0.05 we have enough evidence to reject the null hypothesis and then the best conclusion would be:
B) Since p <a, we conclude that this data shows that seat belts are effective in reducing fatalities.
Explanation:
We have the following info given from the problem
 number of people killed with occupants not wering seat belts
number of people killed with occupants not wering seat belts
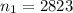 number of people not wearing seat belts
number of people not wearing seat belts
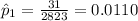 represent the estimated proportion of people killed not wearing seatbelts
represent the estimated proportion of people killed not wearing seatbelts
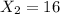 number of people killed with occupants using wering seat belts
number of people killed with occupants using wering seat belts
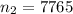 number of people using wearing seat belts
number of people using wearing seat belts
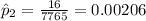 represent the estimated proportion of people killed wearing seatbelts
represent the estimated proportion of people killed wearing seatbelts
We want to check if seat belts are effective in reducing fatalities, so we want to test the following system of hypothesis:
Null hypothesis:
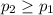
Alternative hypothesis:
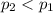
The statistic for this case is given by:
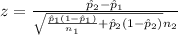
And replacing we got:
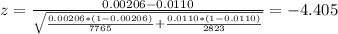
Now we can find the p value since we are using a left tailed test the p value would be:
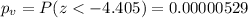
Since the p value is very low compared to the significance level of 0.05 we have enough evidence to reject the null hypothesis and then the best conclusion would be:
B) Since p <a, we conclude that this data shows that seat belts are effective in reducing fatalities.