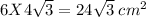Question

Helpppppp

3. Use the diagram of a REGULAR HEXAGON and follow these steps to solve for the area of a hexagon with
sides equal to 4 cm. Leave radicals in your answers
How many equilateral triangles are there?
f we cut an equilateral down the middle (green line) what special triangle do you create?
Sketch and label all side lengths of the 30-60-90 triangle by itself below.
Vhat is the length of the short leg of one 30-60-90 triangle?
Vhat is the length of the hypotenuse of one 30-60-90 triangle?
pply properties of 30-60-90 triangles to calculate the long leg:
That is the vocabulary word for the long side of the 30-60-90 called in the polygon (green line)?
That is the height of the equilateral triangle?
pply triangle area formula to calculate the area of one equilateral triangle:
alculate the area of the complete hexagon by multiplying area of one equilateral triangle by # of triangles:

Answer 1

(a)6 Equilateral Triangles.

(b)30-60-90 triangle.

(c)Attached

(d)Length of the short leg = 2cm.

(e)Length of the hypotenuse= 4cm.

(f)Length of the long leg
`=2√(3)`

(g)Apothem.

(h)Area of One Equilateral triangle
`=4√(3)\:cm^2`

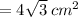
(i)Area of Hexagon =
`=24√(3)\:cm^2`

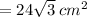
Explanation:

(a)There are 6 Equilateral Triangles.

(b)If we cut an equilateral down the middle (green line), we get a 30-60-90 triangle.

(c)Triangle Attached in 3rd diagram.

(d)The length of the short leg of one of the 30-60-90 triangle is 2cm.

(e)The length of the hypotenuse of one of the 30-60-90 triangle is 4cm.

(f)Length of the long leg

`4^2=2^2+x^2\\x^2=4^2-2^2=12\\x=√(12)\\ x=2√(3)`

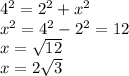
(g)The vocabulary word for the long side of the 30-60-90 called in the polygon (green line) is Apothem.

(h)Area of One Equilateral triangle

Base =4 cm, Height =
`2√(3) cm`

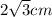
Area

`=0.5X4X2√(3)\\=4√(3)\:cm^2`

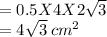
(i)Area of Hexagon =Area of One Equilateral Triangle X 6

=
`6X4√(3)=24√(3)\:cm^2`