Question

In performing a hypothesis test where the null hypothesis is that the population mean is 4.8 against the alternative hypothesis that the population mean is not equal to 4.8, a random sample of 25 items is selected. The sample mean is 4.1 and the sample standard deviation is 1.4. It can be assumed that the population is normally distributed. The degrees of freedom associated with this are _______.

Answer 1

`df=n-1=25-1=24`

Explanation:

Information given

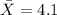`\bar X=4.1` represent the sample mean

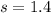`s=1.4` represent the sample standard deviation

`n=25` sample size selected

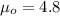`\mu_o =4.8` represent the value to test

System of hypothesis

We are interested in performing a hypothesis test where the null hypothesis is that the population mean is 4.8 against the alternative hypothesis that the population mean is not equal to 4.8, the system of hypothesis would be:

Null hypothesis:
`\mu = 4.8`

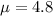
Alternative hypothesis:
`\mu \\eq 4.8`

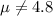
The statistic for this case is given by:

`t=(\bar X-\mu_o)/((s)/(√(n)))` (1)

And the degrees of freedom for this case are:

`df=n-1=25-1=24`