Question

billy bought a new camaro for $35000, but it depreciates in value by 9% a year. What will be the value in 4 years?

Answer 1

$24,001.2

Explanation:

Since it depreciates in value by 9% a year, each year you only have

100% - 9% = 91% of the previous year value.

In this type of problems the best thing is to write an equation:

`value=OriginalValue*(percentageLeft)^(time)`

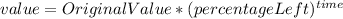
thus, in this case our original value is $35,000

the percentage we have left for the value of the car each year is the 91% (because it depreciates 9%) which we can represent as 0.91, so we have the following equation to calculate the value of the car:

`value=35,000(0.91)^t`

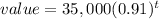
where t is the time in years.

Thus, in 4 years:

`value=35,000(0.91)^(4)\\value=35,000(0.6867)\\value=24,001.2`

the value in 4 years will be $24,001.2