Question

Two identical disks, with rotational inertia I (= 1/2 MR2), roll without slipping across a horizontal floor and then up inclines. Disk A rolls up its incline without sliding. On the other hand, disk B rolls up a frictionless incline. Otherwise the inclines are identical. Disk A reaches a height 12 cm above the floor before rolling down again. Disk B reaches a height above the floor of:

Answer 1

8cm

Step-by-step explanation:

Here, two disc are identical and rolling on the horizontal surface

Also,while disc is in rolling motion its kinetic energy is sum of rotational kinetic energy and transnational kinetic energy.

Therefore,

KE =
`(1)/(2)`mv²+
`(1)/(2)`
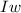`Iw`²

For pure rolling of disc we have:
`v=Rw`

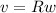
`I=(1)/(2) mR`²

By substituting in KE eq, we get

KE =
`(1)/(2)`mv²+
`(1)/(2)`(
`(1)/(2) mR`²)(
`(v^(2) )/(R^(2) )`)

KE=
`(1)/(2) mv^(2) + (1)/(4) mv^(2)`

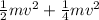
The total kinetic energy will convert into gravitational potential energy when disc roll over the inclined surface.

mgH=
`(1)/(2) mv^(2) + (1)/(4) mv^(2)` =>
`(3)/(4) mv^(2)`

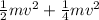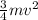
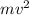`mv^(2)`= 4/3mgH

If another disc rolls up on frictionless inclined plane then it will lose all its translational kinetic energy but rotational kinetic energy will remain as it is as there is no torque on the disc

Therefore, mgh= 1/2mv²

1/2mv²= 4/3mgH

mgh=2/3mgH

h=2/3H

Height = 12cm is given

h= 8cm

Thus, Disk B reaches a height of 8cm above the floor.