Question

The use of social networks has grown dramatically all over the world. In a recent sample of 24 American social network users and each was asked for the amount of time spent (in hours) social networking each day. The mean time spent was 3.19 hours with a standard deviation of 0.2903 hours. Find a 99% confidence interval for the true mean amount of time Americans spend social networking each day

Answer 1

The 99% confidence interval for the true mean amount of time Americans spend social networking each day is (3.02 hours, 3.36 hours).

Explanation:

The (1 - α)% confidence interval for population mean when the population standard deviation is not known is:

`CI=\bar x\pm t_(\alpha/2, (n-1))* (s)/(√(n))`

The information provided is:

`n=24\\\bar x=3.19\ \text{hours}\\s=0.2903\ \text{hours}`

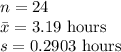
Confidence level = 99%.

Compute the critical value of t for 99% confidence interval and (n - 1) degrees of freedom as follows:

`t_(\alpha/2, (n-1))=t_(0.01/2, (24-1))=t_(0.005, 23)=2.807`

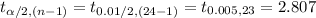
*Use a t-table.

Compute the 99% confidence interval for the true mean amount of time Americans spend social networking each day as follows:

`CI=\bar x\pm t_(\alpha/2, (n-1))* (s)/(√(n))`

`=3.19\pm 2.807* (0.2903)/(√(24))\\\\=3.19\pm 0.1663\\\\=(3.0237, 3.3563)\\\\\approx (3.02, 3.36)`

Thus, the 99% confidence interval for the true mean amount of time Americans spend social networking each day is (3.02 hours, 3.36 hours).