Answer:
a)
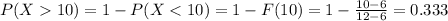
b)
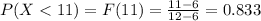
c)
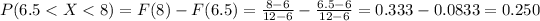
In the figure attached we have the illustration for the probability for each part.
Explanation:
For this case we define the random variable X as the voltages in a circuit and the distribution for X is given by:
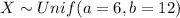
Part a
we want this probability:
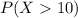
We can use the cumulative distirbution function given by:
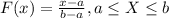
And using the complement rule we have this:
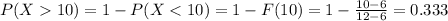
Part b
We want this probability:
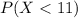
And using the cumulative distirbution function we got:
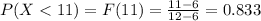
Part c
We want this probability:
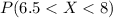
And using the cumulative distirbution function we got:
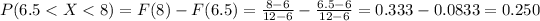
In the figure attached we have the illustration for the probability for each part.